From what I can tell, today is a very special day in certain communities. Apparently, it is “pi” day.
Ooh, it’s pi day already?
You actually know about this holiday?
Of course! It’s 3/14. Like “Pi”, one of the favoritist Greek letters of mathematicians! Stands for 3.1415926…
In fact, I composed a couple of poems about it.
That sounds… really scary.
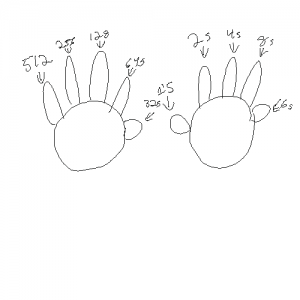
What? It’s fun! In my first one, the number of words in the line maps to the digits of pi. In the second, the number of syllables does.
Want to read them?
No.
Well, you’re going to anyways.
“Pi”em 1
Pie. How delicious.
What?
Not the edible kind?
Really?
What, then, do you mean?
Are you sure you’re not in error about this?
Quite sure?
Well, what kind of pie, then?
The mathematical type of “pi”?
That sounds fun.
Maybe even poem worthy. Wait…
Isn’t that what I have done with this structure?“Pi”em 2
Pie is good.
Yup.
Even math’s pi.
See?
You can make poems!
The syllables map to pi’s digits.
Really!
Isn’t that sort of cool?
See, that wasn’t so bad, was it?
Do you really want me to answer that?
Anyways, readers, are you doing anything special for Pi day?